the Creative Commons Attribution 4.0 License.
the Creative Commons Attribution 4.0 License.
The effect of soil properties on zinc lability and solubility in soils of Ethiopia – an isotopic dilution study
Abdul-Wahab Mossa
Dawd Gashu
Martin R. Broadley
Sarah J. Dunham
Steve P. McGrath
Elizabeth H. Bailey
Scott D. Young
Zinc (Zn) deficiency is a widespread nutritional problem in human populations, especially in sub-Saharan Africa (SSA). The Zn concentration of crops consumed depends in part on the Zn status of the soil. Improved understanding of factors controlling the phyto-availability of Zn in soils can contribute to potential agronomic interventions to tackle Zn deficiency, but many soil types in SSA are poorly studied.
Soil samples (n=475) were collected from a large part of the Amhara Region of Ethiopia, where there is widespread Zn deficiency. Zinc status was quantified by measuring several fractions, including the pseudo-total (aqua regia digestion; ZnTot), available (DTPA (diethylenetriamine pentaacetate) extractable; ZnDTPA), soluble (dissolved in 0.01 M Ca(NO3); ZnSoln) and isotopically exchangeable Zn, using the enriched stable Zn isotope 70Zn (ZnE). Soil geochemical properties were assessed for their influence on Zn lability and solubility. A parameterized geochemical assemblage model (Windermere Humic Aqueous Model – WHAM) was also employed to predict the solid phase fractionation of Zn in tropical soils rather than using sequential chemical extractions.
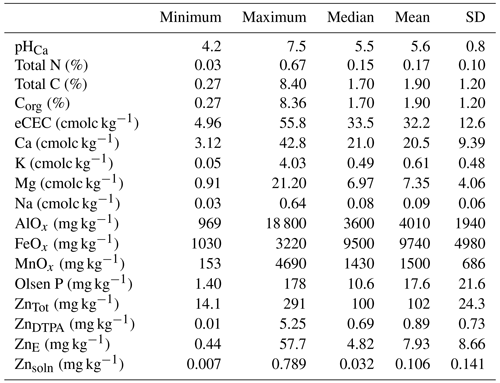
ZnTot ranged from 14.1 to 291 mg kg−1 (median = 100 mg kg−1), whereas ZnDTPA in the majority of soil samples was less than 0.5 mg kg−1, indicating widespread phyto-available Zn deficiency in these soils. The labile fraction of Zn in soil (ZnE as % ZnTot) was low, with median and mean values of 4.7 % and 8.0 %, respectively. Labile Zn partitioning between the solid and the solution phases of soil was highly pH dependent, where 94 % of the variation in the partitioning coefficient of 70Zn was explained by soil pH. Similarly, 86 % of the variation in ZnSoln was explained by soil pH.
Zinc distribution between adsorbed ZnE and ZnSoln was controlled by pH. Notably, Zn isotopic exchangeability increased with soil pH. This contrasts with literature on contaminated and urban soils and may arise from covarying factors, such as contrasting soil clay mineralogy across the pH range of the soils used in the current study. These results could be used to improve agronomic interventions to tackle Zn deficiency in SSA.
- Article
(4016 KB) - Full-text XML
-
Supplement
(488 KB) - BibTeX
- EndNote
Zinc deficiency is a widespread nutritional disorder affecting ∼17 % of the global population and rising to 25 % of the population in countries within sub-Saharan Africa (SSA; Kumssa et al., 2015; Wessells and Brown, 2012). Several interlinked causes contribute to the prevalence of Zn deficiency issues in SSA, including lack of access to animal source foods. This can lead to inadequate Zn intake if the diet is heavily reliant on staple crops, which are inherently low in mineral micronutrients (Joy et al., 2014; Kumssa et al., 2015). Soil degradation and a lack of access to micronutrient fertilizers can contribute to the production of staple crops with poor nutritional quality (Kihara et al., 2020). A total of three-quarters of the arable land in SSA is reported to be depleted in plant nutrients and low in fertility (Toenniessen et al., 2008). However, trace metal dynamics in SSA soils are rarely studied. For instance, we conducted a simple search on Web of Science database using the key words “zinc solubility” and “soil” between 2010 and 2021. The search yielded 24 publications, none of which involved SSA soils. This is potentially a serious omission because Zn geochemistry in SSA soils is likely to differ from that in temperate soils because of differences in geo-colloidal mineralogy, organic C content and the soil pH at which agriculture is practiced.
Phyto-availability of Zn in soil is largely controlled by a dynamic equilibrium between the solid phase and pore water and the absorption mechanisms of plant roots (Groenenberg et al., 2010; Menzies et al., 2007; Peng et al., 2020). Traditionally, chemical extraction procedures used to estimate an assumed phyto-available pool of soil Zn have included reagents which vary widely in extraction power, such as water, neutral salt solutions, dilute strong acids and chelating agents, such as ethylenediaminetetraacetic acid (EDTA) and diethylenetriaminepentaacetic acid (DTPA; Kim et al., 2015). However, these approaches cannot fully characterize both the quantity of potentially available Zn in the soil solid phase and its intensity in the soil solution phase – both of which contribute to the phyto-availability of Zn over the course of a growing season. Isotopic dilution assays may provide a more mechanistically based characterization of the geochemically reactive fraction of Zn in soils which buffers the free ion activity in the soil solution phase (Guzman-Rangel et al., 2020; Hamon et al., 2008; Young et al., 2005). This approach has been extensively used to study contaminated soils (Degryse et al., 2011; Izquierdo et al., 2013; Mossa et al., 2020; Nolan et al., 2005), but its application to Zn in agricultural soils generally, and especially in the soils of SSA countries, is very limited.
The aim of the present study was to investigate the status of Zn lability in soils from a large part of the Amhara Region of Ethiopia, which represent a diverse range of soil types from SSA (Gashu et al., 2020), in which Zn deficiency is thought to be widespread (Hengl et al., 2017). The study used several assays of soil Zn status, including an isotopic dilution assay, employing enriched 70Zn, to examine the soil properties that control Zn phyto-availability. The primary objectives were (i) to determine isotopically exchangeable Zn in soils from the Amhara Region, (ii) to compare different assays of Zn status, (iii) to examine the influence of soil properties on Zn partitioning between the solid and solution phases of these soils and (iv) to investigate the best of three possible input parameters defining reactive Zn tropical soils (isotopically exchangeable, DTPA extractable and total) when using a parameterized geochemical assemblage model to predict Zn solubility – assumed likely to be the principal driver for plant uptake.
2.1 Soil sampling
Field sampling is described in detail in Gashu et al. (2020). Briefly, topsoil was collected from a target of 475 locations in the Amhara Region of Ethiopia (Fig. 1) according to a geospatial design intended to explore spatial variation in soil and crop properties. The sample frame was constrained to sites where the probability that the land was in agricultural use was ≥0.9. At each sampling location, five sub-samples of topsoil were collected from a 100 m2 circular plot, using a Dutch auger with a flight length of 150 mm and a diameter of 50 mm. Any plant material was removed, and the five sub-samples were combined, oven-dried at 40 ∘C for 24–48 h, depending on the moisture content of the soil samples, sieved to <2 mm and homogenized prior to analysis.
2.2 Geochemical analysis
Soluble trace and major metallic elements (MSoln) were determined in the solution phase of soil suspensions in 0.01 M Ca(NO3)2(1:10 soil : solution ratio), following equilibration for 4 d on an end-over-end shaker. The pH of this soil suspension (pHCa) was determined, and then solutions were isolated by centrifugation and filtration (<0.22 µm) prior to elemental analysis by ICP-MS (iCAP Q; Thermo Fisher Scientific, Bremen, Germany). The main parameters of ICP-MS are presented in Table S1 in the Supplement. Total carbon content was determined by dry combustion (Tiessen et al., 1981) using a LECO TruMac CN combustion analyser, and inorganic C was measured using an inorganic carbon analyser (Primacs; Skalar Analytical BV, Breda, the Netherlands). Dissolved organic carbon (DOC) was estimated by measuring non-purgeable organic carbon using a Shimadzu TOC-VWP (total organic carbon and virtual wavelength path) analyser (Shimadzu Corporation, Kyoto, Japan). Estimates of amorphous and poorly crystalline oxides were obtained, following the extraction, with a mixture of ammonium oxalate and oxalic acid at a 1:100 of soil : solution suspension (Schwertmann, 1964). Samples were shaken in the dark at 20 ∘C for 4 h on a reciprocal shaker (120 rpm), then filtered (Whatman no. 42), diluted and acidified to 5 % HNO3 and analysed using inductively coupled plasma optical emission spectrometry (ICP-OES; PerkinElmer Life and Analytical, Shelton, Connecticut, USA). The effective cation exchange capacity (eCEC) was determined using the cobalt hexamine (Cohex) method (ISO 23470; 2018). DTPA-extractable zinc (ZnDTPA) was solubilized by shaking 5 g soil with 10 mL of 0.005 M DTPA, 0.1 M triethanolamine (TEA) and 0.01 M CaCl2 at pH = 7.3 for 2 h on an end-over-end shaker (Lindsay and Norvell, 1978). The soil suspensions were then centrifuged and filtered (<0.22 µm) prior to analysis using inductively coupled plasma mass spectrometry (ICP-MS; iCAP Q; Thermo Fisher Scientific, Bremen, Germany). The pseudo-total Zn concentration in soil (ZnTot) was determined after digesting finely ground soil sample with aqua regia (Crosland et al., 1995) and analysis using ICP-OES. Blanks and in-house standards were included in each extraction run, and calibration standards were checked using independent certified calibration verification standard solutions. Soil reference material ISE 962 purchased from Wageningen Evaluating Programmes for Analytical Laboratories (WEPAL) and was used to confirm the reliability of the aqua regia extractions. Recovery of Zn was 91.3 % ± 2.35 %. Repeat extractions and analysis were undertaken on 10 % of the samples.
2.3 Isotopic dilution assays
To determine the concentration of isotopically exchangeable Zn, 2.0 g of soil was equilibrated with 20 mL of 0.01 M Ca(NO3)2 on an end-over-end shaker for 24 h. This was followed by the addition of 0.4 mL solution with a 70Zn concentration of 11.9 mg L−1, which provided an amount of 70Zn equivalent to 2.3 % of the average ZnTot (104 mg kg−1) concentration in soil. The isotopic tracer solution was prepared from a stock solution enriched with 70Zn (250 mg L−1; isotopic abundance (IA) = 95.47 %). To avoid acidifying the soil suspension, the pH of the spiking solution was adjusted to pH 4.0–4.5 using an ammonium acetate buffer immediately before use. After spiking with the isotope, samples were re-equilibrated for a further 3 d, then centrifuged (3500 rpm for 15 min), filtered (<0.22 µm) and acidified to 2 % HNO3 prior to isotopic analysis using ICP-MS (iCAP Q; Thermo Fisher Scientific, Bremen, Germany). The instrument was operated in collision cell mode, using He with kinetic energy discrimination (KED). Rhodium (103Rh; 10 µg L−1) was used as an internal standard to correct for instrumental drift. The ICP-MS was calibrated for individual Zn isotopes (66Zn and 70Zn) using a multi-isotope Zn standard (CLMS2; SPEX CertiPrep). In practice, it was found that the measurement of 70Zn in the supernatant solution of the soil suspensions required two corrections due to significant, and variable, concentrations of soil-derived 70Ge+ and (plasma-generated) doubly charged (). The correction for 70Ge (IA = 69.9 %) was achieved by determining the intensity (count per second – CPS) of 72Ge in samples and using the measured CPS ratio for Ge standards to infer the proportion of the intensity at 70 arising from 70Ge. The universal isotopic ratio is 1.34; the measured intensity ratio in a Ge standard (including error due to mass discrimination) was typically 1.53. The correction for doubly charged was implemented by running Ce standards, which typically produced a intensity ratio of 0.025, and measuring 140 on samples. The Ce standards were analysed in three concentrations of NaCl (0, 1 and 10 mg L−1) to confirm minimal change in the generation of doubly charged Ce in the plasma with alkali cation concentration. The correction for 70Ge produced a change in ZnE that ranged from 0.027 to 0.976 mg kg−1 (median = 0.253 mg kg−1), while for , the change was 0.024 to 0.973 mg kg−1 (median = 0.747 mg kg−1).
The E value of Zn (ZnE; mg kg−1) was calculated from Eq. (1) as follows:
where ZnSoln is the measured concentration of Zn of an equilibrated soil suspension, the liquid to solid ratio (micromoles per kilogram – µmol L−1), KdLab is the distribution coefficient (micromoles per litre – µmol L−1) of the added 70Zn isotope spike between a weight of soil (S; kilograms) and volume of liquid (L) and is calculated as shown in Eq. (2).
The variable 70Znads is the adsorbed 70Zn spike (micromoles per litre – µmol L−1) and was calculated as the difference between the total 70Zn added to the soil suspension and the amount of 70Zn spike remaining in the solution after equilibration. 70ZnSoln is the measured concentration (micromoles per litre – µmol L−1) of the 70Zn spike in the solution after equilibration. Crucially, the value of 70ZnSoln was corrected for the presence of native 70Zn in the solution, which was estimated from the measured concentration of 66Zn and the known isotopic ratio 70Zn:66Zn; this was implemented after all analytical corrections (70Ge and ) and calculation of the Zn isotope concentrations (micromoles per litre – µmol L−1) from isotopic calibration. The measured 70Zn was overwhelmingly (97.7 % ± 2.20 %) dominated by the added spike. Therefore, any deviation from the expected isotopic ratio of 70Zn:66Zn in the native soil Zn of individual samples would incur a negligible error.
2.4 Geochemical modelling using the Windermere Humic Aqueous Model (WHAM7)
The geochemical model Windermere Humic Aqueous Model (WHAM7; Tipping, 1994) was used to speciate Zn in the solution phase of the 0.01 M Ca(NO3)2 suspensions. Inputs to the model included solution concentrations of cations (Na, Mg, Al, K, Ca, Mn, Fe, Co, Ni, Cu, Zn, Cd, Ba, Pb and U) and the anions (; ) in the solution phase of the Ca(NO3)2 soil suspensions, colloidal (dissolved) fulvic acid, pH, temperature and partial pressure of CO2. The oxide phases Al(OH)3 and Fe(OH)3 were allowed to precipitate. Colloidal fulvic acid (FA) was estimated from non-purgeable organic carbon (NPOC), assuming (i) a carbon content in FA of 50 % and (ii) that 65 % was active (Lofts et al., 2008). Partial pressure of CO2 (PCO2) and the temperature were set to 0.004 atm and 25 ∘C, respectively. WHAM7 was also used to predict the labile pool of Zn (ZnE) within the soil particulate phases. This required the inclusion of suspended particulates, calculated from 2 g solid in 20 mL of electrolyte, and included Fe, Al and Mn oxides (estimated by oxalate extraction) and particulate humic acid which was estimated from soil organic C, assuming 50 % is active humic acid. The surface chemistry of oxides is simulated by a surface complexation model (Lofts and Tipping, 1998), which views the oxide surfaces as bearing hydroxyl groups that interact with protons and metal ions. Default values for specific surface areas in WHAM7 were used.
2.5 Data analysis
Data analysis was carried out using R (version 4.0.2) software (R Core Team, 2021). Measured soil properties were related to Zn lability (ZnE) and the labile distribution coefficient of 70Zn (KdLab), using standard least squares regression. Soil variables used in the regression were soil pH (measured in the Ca(NO3)2 suspensions), organic C (%), the sum of the concentration of Al, Fe and Mn in the oxalate extractions (mol kg−1), dissolved organic C (mg L−1) and the effective cation exchange capacity (eCEC; centimoles of positive charge per kilogram – cmolc kg−1). All data were checked for normality using the Shapiro–Wilk normality test and log transformed when necessary.
3.1 General soil properties
Fluvisols, Leptosols, Lixisols, Luvisols and Vertisols are the prominent soil types in the study area (Dewitte et al., 2013). Most measured soil properties varied widely (Table 1). Soil pH ranged from 4.2–7.5, with ca. 70 % soils having pH values below 6.0. The organic carbon (COrg) also varied widely, with a median value of 1.72 % (Table 1). There was a 10-fold variation in eCEC, potentially indicating a large range of Zn binding strength within the studied soils.
To further illustrate the general status of Zn in the soils, histograms of different indices of Zn lability and solubility are presented in Fig. 2. Total concentration of Zn in soil (ZnTot) ranged from 14.1 to 291 mg kg−1. The median value was 100 mg kg−1 (Fig. 2a and Table 1), which is at the top of the range suggested for uncontaminated soils, i.e. 10–100 mg kg−1 (Mertens and Smolders, 2013). Values of ZnE ranged from 0.44 to 57.7 mg kg−1 (median – 4.82 mg kg−1; Table 1 and Fig. 2b). The labile fraction (ZnE as a percent of ZnTot) ranged from 0.75 % to 69.7 %, with median and mean values of 4.66 % and 8.00 %, respectively. These values (percent of ZnE) are lower than those reported for both contaminated and uncontaminated soils (Degryse et al., 2011; Izquierdo et al., 2013; Marzouk et al., 2013b). The distribution of ZnDTPA concentrations were positively skewed (Fig. 2c), with a variation of 0.01–5.25 mg kg−1 (median = 0.69 mg kg−1). Only 31.4 % of samples had ZnDTPA less than 0.5 mg kg−1, indicating that they are potentially Zn deficient (Mertens and Smolders, 2013). Values of ZnDTPA as a percent of ZnTot ranged from 0.013 % to 3.82 %, with a median and mean of 0.690 % and 0.871 %, respectively. There was a significant but weak positive correlation (r=0.25) between COrg and the percent of ZnDTPA, possibly indicating that Zn bound to soil organic matter is in a form that is accessible to DTPA extraction.
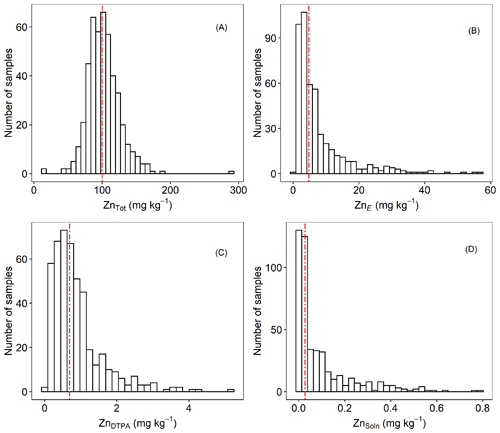
Figure 2Frequency distributions of (a) ZnTot, (b) ZnE, (c) ZnDTPA and (d) ZnSoln concentrations in agricultural topsoil samples from the Amhara Region, Ethiopia. Vertical dashed lines represent median values.
The Zn concentration in 0.01 M Ca(NO3)2, ZnSoln varied by more than 2 orders of magnitude (0.001–0.789 mg kg−1). Values of ZnSoln showed a unimodal and positively skewed distribution (Fig. 2d), indicating predominately small concentrations (<0.1 mg kg−1 in 72 % of soils studied). A maximum of 0.96 % of ZnTot was extracted in Ca(NO3)2 (median = 0.027 %).
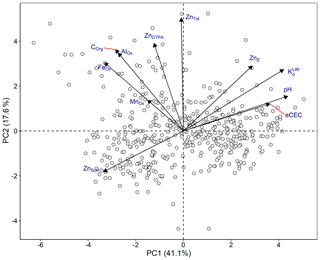
To evaluate the correlation between soil variables, principal component analysis (PCA) and Pearson's correlation analysis were employed (Fig. 3 and Table S2). The first two principal components (PCA 1 and 2) explained 58.7 % of the variation in the data sets; 41.1 % was explained by PCA 1. PCA 1 was strongly correlated with KdLab and soil properties that are likely to affect KdLab, such as pH and eCEC. PCA 2 correlated with ZnDTPA, Corg and mineral oxides (Fig. 3). PCA analysis also shows that ZnSoln and ZnE appear to be inversely correlated, which is mainly a consequence of their opposite trends with pH.
3.2 Isotopically exchangeable Zn
3.2.1 Method assessment and validation
In principle, E-value determination is based on the premise that an added isotope is reversibly adsorbed and is in a dynamic equilibrium between the solid and solution phases (Hamon et al., 2008; Young et al., 2005). Therefore, the reliability of the determined E value rests on an accurate measurement of the distribution coefficient of the added 70Zn (KdLab) and Zn concentration in the soil solution ZnSoln (Eq. 1). For an accurate measurement of KdLab, the added 70Zn must produce a change in the isotopic ratio () that can be reliably quantified while still reflecting the native Zn equilibrium in the soil. As illustrated in Fig. 4a, there was a clear distinction between the natural isotopic ratio (0.02) and measured ratios, with a minimum ratio of 0.15 which is almost 8 times the natural ratio. At the same time, the amount of the added isotope was small compared to ZnTot and amounted to 2.1 % of ZnTot on average (<5 % in 94 % of the samples). To confirm the consistency of ZnSoln, an inter-laboratory comparison was undertaken. Figure 4b shows the results of ZnSoln measurements produced by two different laboratories (University of Nottingham and Rothamsted Research) and using different equilibrating electrolytes (0.01 M Ca(NO3)2 and 0.01 M CaCl2) and different instruments. All data were within 1 order of magnitude from the line of equality, with close agreement (r=0.96) across the range of the ZnSoln concentrations. Thus, given (i) the robustness of the 70Zn distribution coefficient, (ii) the likelihood that the isotopic spike did not substantially affect the native Zn equilibrium or cause precipitation and (iii) the sub-micron filtration step and inter-laboratory agreement for Zn concentration in the 0.01 M Ca electrolyte soil suspensions, we are confident in the validity of the E-value determinations.
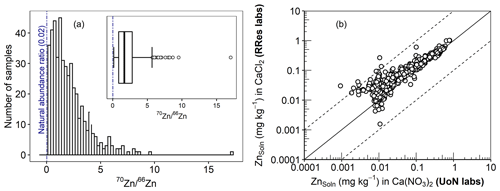
Figure 4(a) Histogram of the isotopic ratio () in the spiked soils. (b) An inter-laboratory comparison of ZnSoln extracted in 0.01 M Ca(NO3)2 at the University of Nottingham (x axis) and in 0.01 CaCl2 at Rothamsted Research (y axis). The dashed lines and the solid line in panel (b) represent 1 log unit interval and the line of equality, respectively.
3.2.2 Soil factors determining Zn lability (ZnE)
Previous studies, mainly on contaminated soils, have reported that the labile fraction of metals tends to decline with rising pH in response to increased adsorption strength (Degryse et al., 2004; Tye et al., 2003); data in the current study showed the opposite trend (Fig. 5a and b). However, with contaminated soils, the behaviour of trace metals often partly reflects the properties of the source of the metal (Mao et al., 2014; Marzouk et al., 2013b). For example, contamination with calcareous materials in the case of soils contaminated with mine spoil produces the co-variation of total Zn concentration with pH. Furthermore, there is usually a restricted pH range in the case of urban soils and temperate agricultural soils. The current study deals with soils that have a comparatively low percent of ZnE and ZnTot and a wide range of pH values (c. 4.0–7.5) which are likely to include substantial differences in geo-colloidal mineralogy (e.g. oxide-based vs. aluminosilicate clays; Fig. 5c). Thus, the trend depicted in Fig. 5a probably reflects a combination of different factors. For example, in soils with higher pH values, it is possible that Zn adsorption is on surfaces which are more likely to retain Zn in a labile form (e.g. humic acid and 2:1 aluminosilicate clays). Similarly, there may be greater Zn fixation under acid conditions because of the greater incidence of oxide-rich mineralogy in highly weathered soils with a low pH (Fig. 5c). A significant negative correlation (; ) between ZnE and the sum of the concentration of mineral oxides in soil may support that hypothesis. Furthermore, solution-phase speciation (from WHAM7) suggested that the proportion of Zn bound to dissolved organic carbon increased with pH (Fig. 6a). At very low Zn concentrations in solution (ZnSoln ca. 1 µg L−1 above pH 6.5), it is possible that the fulvic-bound Zn was sufficiently strongly bound to be non-labile (i.e. excluded from isotopic equilibrium with the added 70Zn). In the calculation of the E value (Eq. 1) this would (erroneously) inflate the apparent ZnE.

Figure 5Concentration of (a) ZnE in soil, (b) ZnE as a percent of ZnTot and (c) mineral oxides in soil as a function of soil pHCa.
A similar outcome would occur if there were significant amounts of non-labile Zn held in particulate form as part of the measurement of ZnSoln at higher pH values. Non-labile particulate metal was first demonstrated by Lombi et al. (2003), who used chelating resin in E-value measurements (Er) to quantify the fraction of the colloidal metal that was not isotopically exchangeable. They reported that Er values were generally less than E values based on equilibrated solution measurements (E), and that the ratio increased with pH. Use of the resin method has produced variable results. Marzouk et al. (2013b) also reported metals associated with sub-micrometre colloidal particles in the solution phase, based on resin phase measurements. They found this association to be positively correlated with soil humus content and pH. However, for their data set, they found that the presence of nano-particulate non-labile Zn in solution produced, on average, less than 2 % difference in the determination of E values (E vs. Er). Mao et al. (2017) also investigated the presence of non-labile metal fractions of Ni, Cu, Zn, Cd and Pb in suspended colloidal particles. They also found an average of only 2 % difference between Er and E for all five metals, and the difference was only significant for Cu with an increased presence of non-labile colloidal particles at high pH – probably organically bound Cu.
If the trend in ZnE with soil pH (Fig. 5a and b) was affected by interferences from particulate materials in the soil solution, then the source of the error would either be in KdLab or ZnSoln – the two variables in the calculation of ZnE (Eq. 1). However, the presence of non-labile Zn within particulate matter in the isolated soil suspension supernatant would not contribute to an error in KdLab. This is because, by definition, the labile spiked isotope is excluded from mixing with the particulate Zn phase. However, the measurement of 66Zn would include Zn in solution and any particulate-bound Zn (<0.22 µm) with which the 70Zn would not have mixed. Thus, it is the determination of ZnSoln (Eq. 1) that may produce an error in ZnE. Tavakkoli et al. (2013) investigated the possible occurrence of non-isotopically exchangeable Zn in sub-micron-sized colloids in filtered soil extraction at high soil pH. They found no non-exchangeable Zn when filtering to <0.1 µm to remove particles but gradually increasing proportions of isotopically non-exchangeable Zn where solutions had been filtered using progressively larger filter pore sizes (0.22, 0.45 and 0.7 µm). In the present study, soil extraction solutions were filtered to <0.22 µm, suggesting that suspended particulates containing non-isotopically exchangeable Zn should be negligible.
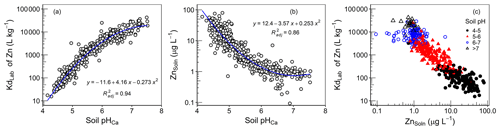
Figure 6Values of (a) KdLab and (b) ZnSoln as a function of soil pH. (c) The relationship between K and ZnSoln.
The possible presence of non-labile nanoparticulate Zn in the soil solution was investigated using a resin (Chelex-100) purification step (Marzouk et al., 2013b) in the determination of ZnE. A comparison was made of ratios in the centrifuged, filtered solution and in a resin extraction of that solution. No evidence of non-labile nanoparticulate Zn below pH 5.5 was found; the isotopic ratios in the solution and resin phases were equal. Unfortunately, at higher soil pH (>5.5), our investigation was confounded due to resin Zn contamination that compromised the measurement of low soluble Zn concentrations in soils with high pH. However, considering the strength of the trend depicted in Fig. 5a, the majority of Zn in the filtered soil solution would have to be present as non-labile particulate matter for the trend shown to be due to non-labile particulate Zn contributing to ZnSoln. This seems unlikely and so we, therefore, suggest that the increase in ZnE values with soil pH in the soils studied is probably a genuine trend.
3.3 Zinc solubility
The partition coefficient in the current study (KdLab) represents the distribution of the added 70Zn spike between the isotopically exchangeable Zn on the solid phase and in the solution phase (Eq. 2). Values of Kdlab varied by more than 3 orders of magnitude – ranging from 15.4 to 42600 L kg−1. As shown in Fig. 6a, values of KdLab were highly pH dependent, in agreement with increased adsorption strength of cationic trace metals onto soil surfaces with pH. Regression analysis of soil properties (eCEC, COrg, ZnTot and mineral oxides) against KdLab is presented in Table 2. Only significant variables were retained in regression equations, and the variables were checked for multicollinearity using variance inflation factors (VIFs). Values of VIFs for all variables were less than 3. While all variables in Table 2 were significant in the regression analysis, they accounted for a very small proportion of the variation (<4 %) in the data. The majority of the variation in the data (90 %) was explained solely by soil pH (Table 2 and Fig. 6a). Despite the fact that soil organic matter is known to be an important sorbent for trace elements (Degryse et al., 2011), COrg had a negligible influence on KdLab.
As seen for KdLab, ZnSoln was also mainly controlled by soil pH; 77 % of the variation in ZnSoln was explained solely by soil pH (Fig. 6b). There was also a weak, but significant, correlation between ZnSoln and soil COrg (r=0.23, ); some influence on metal adsorption strength would be expected because of the importance of humus as a metal adsorbent (Fan et al., 2016). However, the limited effect of soil organic matter may be due to a dual influence on Zn solubility. Soil humus will contribute to Zn adsorption within the soil solid phase but also produce greater DOC (r=0.47 between Corg and DOC), which will promote the dissolved organo-complexation of Zn.
The concentration of Zn in soil solutions is largely determined by the combined influence of soil properties, which affect the strength of adsorption and the total Zn concentration in soil. But, the relationship between KdLab and ZnSoln (Fig. 6c) demonstrates the much greater importance of soil characteristics over the influence of ZnTot in the Amhara soils. In considering the relationship in Fig. 6c, it should be emphasized that KdLab and ZnSoln are completely independent of each other. The value of KdLab is the distribution coefficient of the added 70Zn isotope and ZnSoln is determined from measured values of 66Zn; this negates the common, and justified, criticism of such relations in which ZnSoln is the denominator of the Kd, which would tend to produce a declining trend with ZnSoln. Therefore, the very strong capacity–intensity dependence of the studied soils genuinely reflects control by soil properties over Zn solubility. In particular, for the soils studied, soil pH alone virtually controls the strength of Zn adsorption and ZnSoln (Fig. 6a and b; Table 2), despite considerable variation in ZnTot (14.1–291 mg kg−1; Table 1).
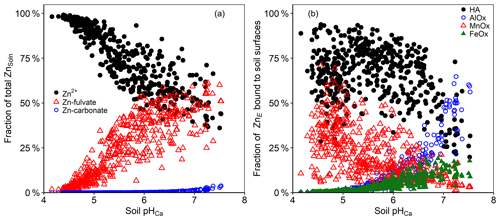
Figure 7(a) Zn speciation in the solution phase of Ethiopian soil suspensions, as predicted by WHAM7 (free divalent ions – black circles; FA complexes – red triangles; carbonate complexes – blue circles). (b) Zn fractionation in the solid phase, as predicted by WHAM7 (HA complexes – black circles; Al oxide bound – blue circles; Mn oxide bound – red triangles; Fe oxide bound – green triangles).
3.4 Multi-surface modelling of soluble Zn concentration
It is widely recognized that, while the total concentration of an element in soil is important, it is the chemical speciation that plays a key role in determining availability to plants. Despite that, direct measurement of the chemical forms of an element is technically challenging. Therefore, geochemical modelling offers a feasible alternative and has been widely applied to soil (Bonten et al., 2008; Cui and Weng, 2015; Klinkert and Comans, 2020).
The WHAM7 predictions of labile Zn distribution among different soil surfaces are presented in Fig. 7b. At low soil pH, the WHAM7 model predicted the sorption to be overwhelmingly onto Mn oxide and humic acids, whereas, at intermediate and high pH, humic-acid-bound Zn became dominant (Buekers et al., 2008; Marzouk et al., 2013b). WHAM7 predicts a negligible role for Fe oxide in adsorbing Zn, but at pH > 6.5, sorption onto Al oxides was important. The fractionation suggested by the WHAM7 model relates only to labile Zn and does not predict the location of the “fixed” (non-labile) Zn in the soils.
As input for the WHAM model, soil reactive organic matter in the current study was estimated from soil organic C, assuming 50 % is active humic acid. However, the composition and the reactivity of soil organic matter will be different from one soil to another; for instance, Van Eynde et al. (2020) found that the average fraction of humic substances in five tropical soils was 36 %. Consequently, there will be an associated error when relying on such assumptions in the absence of a full characterization of soil organic matter because the model was developed based on data derived from purified humic substances. This is particularly important in the current study because, while WHAM has been used and validated in several studies on temperate soils (Buekers et al., 2008; Izquierdo et al., 2013; Mao et al., 2017), to our knowledge, it has not been used on tropical soils, which distinctively have small organic matter content. To test the effect of soil organic matter composition on model performance, we ran the model assuming 36 % of soil organic C to be active humic substances. The results of these simulations are reported in Fig. S1 in the Supplement. The change in ZnSoln was pH dependent, ranged from −4.8 % to −39.8 % and the maximum change occurred at soil pH 5.3. This confirms that accurate inputs to the model are necessary to ensure meaningful modelling of metals in tropical soils.
Both amorphous and crystalline Fe oxide are involved in metal binding in soil. The specific area of the crystalline Fe oxide have been assumed to be one-sixth of that of the amorphous Fe oxide (Dijkstra et al., 2004). However, it was previously found that oxalate extractable Fe (amorphous) was smaller than dithionite extractable Fe (crystalline and amorphous) in tropical soils (Van Eynde et al., 2020). For comparison, model performance was tested using double the amount of amorphous oxides as inputs. The results of these comparisons are presented in Fig. S2. When doubling the amount of particulate Fe oxide in WHAM inputs, there was, on average, 3.42 %, 5.43 % and 2.56 % decrease in predicted ZnSoln when using ZnE, ZnDTPA and ZnTot as inputs, respectively. The change was highly pH dependent, and the effect was negligible at soil pH < 5.5 (Fig. S2).
In the current study, Mn oxides appear to be a very important binding phase for Zn. The binding constant, pKMH, for Zn onto Mn oxide in WHAM7 is 1.6. However, Lofts and Tipping (1998) found that the binding constant for Zn onto Mn oxide ranged from 0.6 to 4.2. The authors attributed the difficulty in modelling adsorption onto Mn oxides to sparse adsorption data, uncertainty in Mn oxide properties and variation among results from experimental data. Therefore, the results of modelling Zn adsorption onto Mn oxides, as predicted by WHAM, should be viewed with caution.
The speciation of Zn in the soil solution, as calculated by WHAM, is presented in Fig. 7a. It was predicted that the free Zn ion activity (Zn2+) constituted 36.1 % to 99.2 % (median = 77.1 %) of the total ZnSoln and was highly correlated with pH. At pH < 5.5, the majority of ZnSoln was present as the free Zn2+ ion (>61 %). This percentage decreased to an average of 49 % at soil pH > 7. Previous studies have also shown minimal complexation of Zn in the soil solution at pH < 6.5 (Catlett et al., 2002; Rutkowska et al., 2015). The proportion of the total Zn present as dissolved fulvic acid complexes ranged from 0.81 % to 61.2 % (median 22.7 %). The proportion of FA-complexed Zn increased with increasing soil pH (Fig. 6a); at pH > 7, an average of 47.7 % of ZnSoln was apparently complexed to FA. The only inorganic Zn complexes were carbonates, and these accounted for <4 % at soil pH > 7.
An important consideration when using geochemical models is the choice of the “reactive” pool of metals, which is in equilibrium with the soil solution, as an input variable. It has been well established that the total concentration of metals does not reflect the reactive fraction in soil (Kelepertzis and Argyraki, 2015; Peng et al., 2018; Rodrigues et al., 2013). Extractions with 0.43 M nitric acid (HNO3) and EDTA have been frequently used to approximate the geochemically reactive pool of metals in soil (Agrelli et al., 2020; Groenenberg et al., 2017; Liu et al., 2019; Ren et al., 2017). However, the isotopic dilution method is recognized to be, conceptually, the most robust and mechanistically based method that reliably quantifies the reactive pool of metals in soil (Groenenberg et al., 2017; Hamon et al., 2008; Peng et al., 2018). To assess the capability of WHAM7 to predict Zn solubility, the concentrations in the solution phase (ZnSoln) were compared with the outputs from fractionation of Zn across the whole soil solution system, using either ZnTot, ZnE or ZnDTPA concentrations as the fraction of Zn controlling Zn solubility. Results of these simulations are presented in Fig. 8.
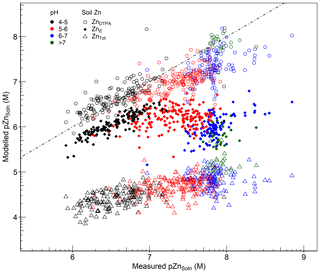
Figure 8Comparison between observed pZnSoln (x axis) and modelled pZnSoln (y axis), using either ZnDTPA, ZnE or ZnTot as WHAM inputs. The dashed line represents the line of equality.
Figure 8 clearly illustrates that using ZnE substantially improves the prediction of Zn solubility compared to using ZnTot, particularly at low soil pH. This reinforces the conclusion that the geochemically reactive metal pool, rather than the total soil Zn concentration, is the most relevant representation of Zn availability in soil. At high pH (>7.5), the model predicts higher ZnSoln than observed concentrations. This may be partly due to limitations in binding surfaces considered in WHAM7. At pH > 7, adsorption on calcium carbonate or phosphate minerals may occur which is not accounted for in WHAM7. This was reported by Peng et al. (2018), who excluded data at pH > 7 from their results when using WHAM7 to predict the solid solution partition and speciation of heavy metals,in response to a lack of consideration of precipitation on carbonates. Izquierdo et al. (2013) listed the failure to include binding to carbonate surfaces as a possible source of error in predicting metal concentration in the soil solution from WHAM7. Mao et al. (2017) also attributed the overestimation of metal concentrations in the soil solution to the exclusion of phases such as calcite and hydroxyapatite as binding phases in WHAM7. Additionally, overestimation of E values at high pH due to the presence of (non-labile) Zn, which has not isotopically mixed with the added 70Zn spike, would also explain the poorer performance of WHAM7 in predicting Zn solubility at high pH. Bonten et al. (2008) also reported overestimation of Zn concentration calculated by the geochemical model ORCHESTRA. They pointed out that some authors had suggested sorption of Zn in Al-layered double hydroxides or phyllosilicates, in soils at near-neutral pH, as a potential reason for overprediction of Zn solubility.
When ZnDTPA was used as input, prediction of Zn solubility by WHAM7 was apparently improved over that achieved by using ZnE (Fig. 8), particularly at high pH. This may confirm the possible overestimation of ZnE, as discussed above. Alternatively, it may reflect counteracting errors between (i) the inadequacy of DTPA (0.005 M) as an extractant which would decrease modelled ZnSoln and (ii) the underestimation of Zn binding in WHAM7 at high pH, which would raise the estimate of ZnSoln. It is recognized, for example, that 0.005 M DTPA extracts less Zn from soil than 0.05 M EDTA and also underestimates ZnE (Marzouk et al., 2013a). To assess whether the binding capacity of the DTPA used was limited, the mole ratios of cations to DTPA in the extracted solutions (excluding alkali alkali–earth cations) were calculated. The average ratio was only 0.17±0.08, suggesting that the DTPA extractant was probably not capacity limited. These results suggest, broadly, that both ZnDTPA and ZnE may be reasonable estimates of the labile pool of Zn in soil. DTPA appears to provide a better estimate of ZnSoln when using a current geochemical model, especially at high pH. Alternatively, the isotopic dilution method, measured in neutral 0.01 M Ca(NO3)2, probably better reflects variation with pH in the labile Zn KdLab value and possibly in the true labile pool of Zn in soil compared with DTPA, which is buffered at pH 7.3.
3.5 Free Zn2+ activity in soil solution
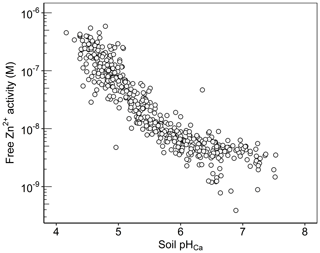
The free ion activity is considered a key factor controlling plant uptake, although other factors will affect buffering and diffusion rates in the soil (Degryse et al., 2012). The concentration of Zn2+ activity in the soil solution is effectively an integration of soil properties that govern sorption processes. Data presented in Fig. 9 show that the activity of Zn2+ is highly pH dependent; 81 % of the variation in the free Zn2+ activity was accounted for solely by soil pH in the Ethiopian Amhara soils. The concentration of free Zn2+ activity varied over 3 orders of magnitude. The range of the free Zn2+ activity is probably a product of the counteracting effects of ZnE and ZnSoln variation with pH; ZnE increases with pH, while ZnSoln falls as pH rises, as discussed above (Figs. 5b and 6b).
In this study, combining (i) an isotopic dilution approach to determining reactive soil Zn (ZnE) and the relative strength of available Zn adsorption (Kd) with (ii) geochemical speciation modelling (WHAM7) provided useful insights into the intrinsic reactivity of Zn in SSA soils at a regional scale and revealed the key soil variables determining Zn solubility. In particular, the results demonstrated that intrinsic soil properties, rather than the variation in ZnTot concentration, were more important in determining the adsorption strength (Kd) of labile Zn and Zn solubility. Specifically, in the Ethiopian Amhara data set, soil pH was the key determining factor. Surprisingly, the traditional DTPA extraction method provided a better estimate of ZnSoln, predicted from a geochemical modelling approach, when compared with ZnE as a model input variable. Reasons for this remain unresolved and may reflect shortcomings in either (i) the crude nature of soil analysis for model inputs or (ii) poorer model prediction at higher pH values due to further soil Zn adsorbents not considered within the WHAM7 model, such as Ca carbonate and phosphate minerals.
These findings may have practical implications for agronomic interventions to improve crop Zn concentrations because they provide a tool for differentiating between soils in terms of both the strength with which they adsorb Zn and their available Zn reservoir. This is an important consideration for a site-specific strategy to ensure more effective agronomic biofortification of staple crops with Zn fertilizers (Joy et al., 2015; Manzeke et al., 2014, 2020; Zia et al., 2020). It may be suggested, for example, that in soils with pH > 6.5 foliar Zn application may be most appropriate because Zn is consistently more strongly adsorbed as pH rises, while in soils with lower pH applying Zn-containing fertilizers to the soil might be feasible. Furthermore, these findings can be used to identify areas where the use of soil management practices, such as liming and organic matter incorporation, are likely to impact Zn availability in soil and, thereby, affect Zn concentration of staple crops (Manzeke et al., 2019; Wood et al., 2018).
Zinc uptake by crop plants will be determined by a combination of (i) soil Zn availability and (ii) plant controls over uptake and transport. It is likely that soil Zn availability will reflect both the solubility and the magnitude of the reactive Zn reservoir that supports Zn2+ ion activity in the soil solution. For the Amhara soils studied, it appears that (i) DTPA-extractable Zn provides the best estimate of the available Zn pool controlling Zn2+ solubility and (ii) that pH is the best predictor of the strength of labile Zn adsorption. Both assays are well recognized and fall within the scope of most agronomic laboratories. It, therefore, seems reasonable to suggest that ZnDTPA and soil pH may, in combination, be tested as predictor variables for Zn uptake by staple crops in considering both crop Zn deficiency and biofortification for human health.
Data used in this study are available from the corresponding author upon reasonable request, as the lab data will be published as part of a national data set.
The supplement related to this article is available online at: https://doi.org/10.5194/soil-7-255-2021-supplement.
Conceptualization of the study for this paper was done by AWM, MRB, SPM and SDY, with input from EHB, DG and SJD. Data curation and investigation were conducted by AWM. Analysis, methodology and visualization for the paper were performed by AWM, with substantial input from MRB, SDY, EHB and feedback from all authors. AWM wrote the initial draft, and all authors were involved in the reviewing and editing of the paper.
The authors declare that they have no conflict of interest.
The funders of this paper were not involved in the study design, the collection, management, analysis and interpretation of data, the writing of the report or the decision to submit the report for publication.
The authors gratefully acknowledge the contribution made to this research by the field sampling team of the Amhara National Regional Bureau of Agriculture, Debebe Hailu and Aregash Beshire, who also contributed to sample preparation, the staff at Rothamsted, who performed many of the extractions and instrumental analyses, and Saul Vazquez Reina at the University of Nottingham, who performed the ICP-MS analysis. The authors would like to thank Diriba Kumssa (University of Nottingham) for providing a map of the sampling area.
This research has been supported by the Biotechnology and Biological Sciences Research Council (grant no. BB/P023126/1) and the Bill and Melinda Gates Foundation (grant no. INV-009129).
This paper was edited by Claudio Zaccone and reviewed by Jan Groenenberg and one anonymous referee.
Agrelli, D., Caporale, A. G., and Adamo, P.: Assessment of the Bioavailability and Speciation of Heavy Metal(loid)s and Hydrocarbons for Risk-Based Soil Remediation, Agronomy, 10, 1440, https://doi.org/10.3390/agronomy10091440, 2020.
Bonten, L. T. C., Groenenberg, J. E., Weng, L., and van Riemsdijk, W. H.: Use of speciation and complexation models to estimate heavy metal sorption in soils, Geoderma, 146, 303–310, https://doi.org/10.1016/j.geoderma.2008.06.005, 2008.
Buekers, J., Degryse, F., Maes, A., and Smolders, E.: Modelling the effects of ageing on Cd, Zn, Ni and Cu solubility in soils using an assemblage model, Eur. J. Soil Sci., 59, 1160–1170, https://doi.org/10.1111/j.1365-2389.2008.01053.x, 2008.
Catlett, K. M., Heil, D. M., Lindsay, W. L., and Ebinger, M. H.: Soil Chemical Properties Controlling Zinc2+ Activity in 18 Colorado Soils, Soil Sci. Soc. Am. J., 66, 1182–1189, https://doi.org/10.2136/sssaj2002.1182, 2002.
Crosland, A. R., Zhao, F. J., McGrath, S. P., and Lane, P. W.: Comparison of aqua regia digestion with sodium carbonate fusion for the determination of total phosphorus in soils by inductively coupled plasma atomic emission spectroscopy (ICP), Commun. Soil Sci. Plant Anal., 26, 1357–1368, https://doi.org/10.1080/00103629509369377, 1995.
Cui, Y. and Weng, L.: Interpretation of heavy metal speciation in sequential extraction using geochemical modelling, Environ. Chem., 12, 163–173, https://doi.org/10.1071/EN13216, 2015.
Degryse, F., Buekers, J., and Smolders, E.: Radio-labile cadmium and zinc in soils as affected by pH and source of contamination, Eur. J. Soil Sci., 55, 113–122, https://doi.org/10.1046/j.1351-0754.2003.0554.x, 2004.
Degryse, F., Voegelin, A., Jacquat, O., Kretzschmar, R., and Smolders, E.: Characterization of zinc in contaminated soils: complementary insights from isotopic exchange, batch extractions and XAFS spectroscopy, Eur. J. Soil Sci., 62, 318–330, https://doi.org/10.1111/j.1365-2389.2010.01332.x, 2011.
Degryse, F., Shahbazi, A., Verheyen, L., and Smolders, E.: Diffusion Limitations in Root Uptake of Cadmium and Zinc, But Not Nickel, and Resulting Bias in the Michaelis Constant, Plant Physiol., 160, 1097–1109, https://doi.org/10.1104/pp.112.202200, 2012.
Dewitte, O., Jones, A., Spaargaren, O., Breuning-Madsen, H., Brossard, M., Dampha, A., Deckers, J., Gallali, T., Hallett, S., Jones, R., Kilasara, M., Le Roux, P., Michéli, E., Montanarella, L., Thiombiano, L., Van Ranst, E., Yemefack, M., and Zougmore, R.: Harmonisation of the soil map of Africa at the continental scale, Geoderma, 211–212, 138–153, https://doi.org/10.1016/j.geoderma.2013.07.007, 2013.
Dijkstra, J. J., Meeussen, J. C. L., and Comans, R. N. J.: Leaching of Heavy Metals from Contaminated Soils: An Experimental and Modeling Study, Environ. Sci. Technol., 38, 4390–4395, https://doi.org/10.1021/es049885v, 2004.
Fan, T.-T., Wang, Y.-J., Li, C.-B., He, J.-Z., Gao, J., Zhou, D.-M., Friedman, S. P., and Sparks, D. L.: Effect of Organic Matter on Sorption of Zn on Soil: Elucidation by Wien Effect Measurements and EXAFS Spectroscopy, Environ. Sci. Technol., 50, 2931–2937, https://doi.org/10.1021/acs.est.5b05281, 2016.
Gashu, D., Lark, R. M., Milne, A. E., Amede, T., Bailey, E. H., Chagumaira, C., Dunham, S. J., Gameda, S., Kumssa, D. B., Mossa, A. W., Walsh, M. G., Wilson, L., Young, S. D., Ander, E. L., Broadley, M. R., Joy, E. J. M., and McGrath, S. P.: Spatial prediction of the concentration of selenium (Se) in grain across part of Amhara Region, Ethiopia, Sci. Total Environ., 733, 139231, https://doi.org/10.1016/j.scitotenv.2020.139231, 2020.
Groenenberg, J. E., Römkens, P. F. a. M., Comans, R. N. J., Luster, J., Pampura, T., Shotbolt, L., Tipping, E., and Vries, W. D.: Transfer functions for solid-solution partitioning of cadmium, copper, nickel, lead and zinc in soils: derivation of relationships for free metal ion activities and validation with independent data, Eur. J. Soil Sci., 61, 58–73, https://doi.org/10.1111/j.1365-2389.2009.01201.x, 2010.
Groenenberg, J. E., Römkens, P. F. A. M., Zomeren, A. V., Rodrigues, S. M., and Comans, R. N. J.: Evaluation of the Single Dilute (0.43 M) Nitric Acid Extraction to Determine Geochemically Reactive Elements in Soil, Environ. Sci. Technol., 51, 2246–2253, https://doi.org/10.1021/acs.est.6b05151, 2017.
Guzman-Rangel, G., Martínez-Villegas, N., and Smolders, E.: The labile fractions of metals and arsenic in mining-impacted soils are explained by soil properties and metal source characteristics, J. Environ. Qual., 49, 417–427, https://doi.org/10.1002/jeq2.20055, 2020.
Hamon, R. E., Parker, D. R., and Lombi, E.: Advances in Isotopic Dilution Techniques in Trace Element Research: A Review of Methodologies, Benefits, and Limitations, in: Advances in Agronomy, vol. 99, Academic Press, Amsterdam, 289–343, https://doi.org/10.1016/S0065-2113(08)00406-9, 2008.
Hengl, T., Leenaars, J. G. B., Shepherd, K. D., Walsh, M. G., Heuvelink, G. B. M., Mamo, T., Tilahun, H., Berkhout, E., Cooper, M., Fegraus, E., Wheeler, I., and Kwabena, N. A.: Soil nutrient maps of Sub-Saharan Africa: assessment of soil nutrient content at 250 m spatial resolution using machine learning, Nutr. Cycl. Agroecosyst., 109, 77–102, https://doi.org/10.1007/s10705-017-9870-x, 2017.
ISO 23470: Soil quality – Determination of effective cation exchange capacity (CEC) and exchangeable cations using a hexamminecobalt (III) chloride solution, 2018.
Izquierdo, M., Tye, A. M., and Chenery, S. R.: Lability, solubility and speciation of Cd, Pb and Zn in alluvial soils of the River Trent catchment UK, Environ. Sci.: Proc. Imp., 15, 1844–1858, https://doi.org/10.1039/C3EM00370A, 2013.
Joy, E. J. M., Ander, E. L., Young, S. D., Black, C. R., Watts, M. J., Chilimba, A. D. C., Chilima, B., Siyame, E. W. P., Kalimbira, A. A., Hurst, R., Fairweather-Tait, S. J., Stein, A. J., Gibson, R. S., White, P. J., and Broadley, M. R.: Dietary mineral supplies in Africa, Physiologia Plantarum, 151, 208–229, https://doi.org/10.1111/ppl.12144, 2014.
Joy, E. J. M., Stein, A. J., Young, S. D., Ander, E. L., Watts, M. J., and Broadley, M. R.: Zinc-enriched fertilisers as a potential public health intervention in Africa, Plant Soil, 389, 1–24, https://doi.org/10.1007/s11104-015-2430-8, 2015.
Kelepertzis, E. and Argyraki, A.: Geochemical associations for evaluating the availability of potentially harmful elements in urban soils: Lessons learnt from Athens, Greece, Appl. Geochem., 59, 63–73, https://doi.org/10.1016/j.apgeochem.2015.03.019, 2015.
Kihara, J., Bolo, P., Kinyua, M., Rurinda, J., and Piikki, K.: Micronutrient deficiencies in African soils and the human nutritional nexus: opportunities with staple crops, Environ. Geochem. Health, 42, 3015–3033, https://doi.org/10.1007/s10653-019-00499-w, 2020.
Kim, R.-Y., Yoon, J.-K., Kim, T.-S., Yang, J. E., Owens, G., and Kim, K.-R.: Bioavailability of heavy metals in soils: definitions and practical implementation – a critical review, Environ. Geochem. Health, 37, 1041–1061, https://doi.org/10.1007/s10653-015-9695-y, 2015.
Klinkert, S. and Comans, R. N. J.: Geochemical Multisurface Modeling of Reactive Zinc Speciation in Compost as Influenced by Extraction Conditions, Environ. Sci. Technol., 54, 2467–2475, https://doi.org/10.1021/acs.est.9b04104, 2020.
Kumssa, D. B., Joy, E. J. M., Ander, E. L., Watts, M. J., Young, S. D., Walker, S., and Broadley, M. R.: Dietary calcium and zinc deficiency risks are decreasing but remain prevalent, Scient. Rep., 5, 10974, https://doi.org/10.1038/srep10974, 2015.
Lindsay, W. L. and Norvell, W. A.: Development of a DTPA Soil Test for Zinc, Iron, Manganese, and Copper, Soil Sci. Soc. Am. J., 42, 421–428, https://doi.org/10.2136/sssaj1978.03615995004200030009x, 1978.
Liu, P., Wang, P., Lu, Y., Ding, Y., Lu, G., Dang, Z., and Shi, Z.: Modeling kinetics of heavy metal release from field-contaminated soils: Roles of soil adsorbents and binding sites, Chem. Geol., 506, 187–196, https://doi.org/10.1016/j.chemgeo.2018.12.030, 2019.
Lofts, S. and Tipping, E.: An assemblage model for cation binding by natural particulate matter, Geochim. Cosmochim. Ac., 62, 2609–2625, https://doi.org/10.1016/S0016-7037(98)00183-5, 1998.
Lofts, S., Tipping, E., and Hamilton-Taylor, J.: The Chemical Speciation of Fe(III) in Freshwaters, Aquat. Geochem., 14, 337–358, https://doi.org/10.1007/s10498-008-9040-5, 2008.
Lombi, E., Hamon, R. E., McGrath, S. P., and McLaughlin, M. J.: Lability of Cd, Cu, and Zn in Polluted Soils Treated with Lime, Beringite, and Red Mud and Identification of a Non-Labile Colloidal Fraction of Metals Using Isotopic Techniques, Environ. Sci. Technol., 37, 979–984, https://doi.org/10.1021/es026083w, 2003.
Manzeke, G. M., Mtambanengwe, F., Nezomba, H., and Mapfumo, P.: Zinc fertilization influence on maize productivity and grain nutritional quality under integrated soil fertility management in Zimbabwe, Field Crops Res., 166, 128–136, https://doi.org/10.1016/j.fcr.2014.05.019, 2014.
Manzeke, M. G., Mtambanengwe, F., Watts, M. J., Hamilton, E. M., Lark, R. M., Broadley, M. R., and Mapfumo, P.: Fertilizer management and soil type influence grain zinc and iron concentration under contrasting smallholder cropping systems in Zimbabwe, Scient. Rep., 9, 6445, https://doi.org/10.1038/s41598-019-42828-0, 2019.
Manzeke, M. G., Mtambanengwe, F., Watts, M. J., Broadley, M. R., Murray, L. R., and Mapfumo, P.: Nitrogen effect on zinc biofortification of maize and cowpea in Zimbabwean smallholder farms, Agron. J., 112, 2256–2274, https://doi.org/10.1002/agj2.20175, 2020.
Mao, L., Bailey, E. H., Chester, J., Dean, J., Ander, E. L., Chenery, S. R., and Young, S. D.: Lability of Pb in soil: effects of soil properties and contaminant source, Environ. Chem., 11, 690–701, https://doi.org/10.1071/EN14100, 2014.
Mao, L. C., Young, S. D., Tye, A. M., and Bailey, E. H.: Predicting trace metal solubility and fractionation in Urban soils from isotopic exchangeability, Environ. Pollut., 231, 1529–1542, https://doi.org/10.1016/j.envpol.2017.09.013, 2017.
Marzouk, E. R., Chenery, S. R., and Young, S. D.: Measuring reactive metal in soil: a comparison of multi-element isotopic dilution and chemical extraction, Eur. J. Soil Sci., 64, 526–536, https://doi.org/10.1111/ejss.12043, 2013a.
Marzouk, E. R., Chenery, S. R., and Young, S. D.: Predicting the solubility and lability of Zn, Cd, and Pb in soils from a minespoil-contaminated catchment by stable isotopic exchange, Geochim. Cosmochim. Ac., 123, 1–16, https://doi.org/10.1016/j.gca.2013.09.004, 2013b.
Menzies, N. W., Donn, M. J., and Kopittke, P. M.: Evaluation of extractants for estimation of the phytoavailable trace metals in soils, Environ. Pollut., 145, 121–130, https://doi.org/10.1016/j.envpol.2006.03.021, 2007.
Mertens, J. and Smolders, E.: Zinc, in: Heavy Metals in Soils: Trace Metals and Metalloids in Soils and their Bioavailability, edited by: Alloway, B. J., Springer Netherlands, Dordrecht, 465–493, https://doi.org/10.1007/978-94-007-4470-7_17, 2013.
Mossa, A.-W., Young, S. D., and Crout, N. M. J.: Zinc uptake and phyto-toxicity: Comparing intensity- and capacity-based drivers, Sci. Total Environ., 699, 134314, https://doi.org/10.1016/j.scitotenv.2019.134314, 2020.
Nolan, A. L., Zhang, H., and McLaughlin, M. J.: Prediction of Zinc, Cadmium, Lead, and Copper Availability to Wheat in Contaminated Soils Using Chemical Speciation, Diffusive Gradients in Thin Films, Extraction, and Isotopic Dilution Techniques, J. Environ. Qual., 34, 496–507, https://doi.org/10.2134/jeq2005.0496, 2005.
Peng, Q., Wang, D., Wang, M., Zhou, F., Yang, W., Liu, Y., and Liang, D.: Prediction of selenium uptake by pak choi in several agricultural soils based on diffusive gradients in thin-films technique and single extraction, Environ. Pollut., 256, 113414, https://doi.org/10.1016/j.envpol.2019.113414, 2020.
Peng, S., Wang, P., Peng, L., Cheng, T., Sun, W., and Shi, Z.: Predicting Heavy Metal Partition Equilibrium in Soils: Roles of Soil Components and Binding Sites, Soil Sci. Soc. Am. J., 82, 839–849, https://doi.org/10.2136/sssaj2018.03.0104, 2018.
R Core Team: R: A language and environment for statistical computing, R Foundation for Statistical Computing, Vienna, Austria, available at: https://www.R-project.org/, last access: 24 April 2021.
Ren, Z., Sivry, Y., Tharaud, M., Cordier, L., Li, Y., Dai, J., and Benedetti, M. F.: Speciation and reactivity of lead and zinc in heavily and poorly contaminated soils: Stable isotope dilution, chemical extraction and model views, Environ. Pollut., 225, 654–662, https://doi.org/10.1016/j.envpol.2017.03.051, 2017.
Rodrigues, S. M., Cruz, N., Coelho, C., Henriques, B., Carvalho, L., Duarte, A. C., Pereira, E., and Römkens, P. F. A. M.: Risk assessment for Cd, Cu, Pb and Zn in urban soils: Chemical availability as the central concept, Environ. Pollut., 183, 234–242, https://doi.org/10.1016/j.envpol.2012.10.006, 2013.
Rutkowska, B., Szulc, W., Bomze, K., Gozdowski, D., and Spychaj-Fabisiak, E.: Soil factors affecting solubility and mobility of zinc in contaminated soils, Int. J. Environ. Sci. Technol., 12, 1687–1694, https://doi.org/10.1007/s13762-014-0546-7, 2015.
Schwertmann, U.: Differenzierung der Eisenoxide des Bodens durch Extraktion mit Ammoniumoxalat-Lösung, Zeitschrift für Pflanzenernährung, Düngung, Bodenkunde, 105, 194–202, https://doi.org/10.1002/jpln.3591050303, 1964.
Tavakkoli, E., Donner, E., Juhasz, A., Naidu, R., and Lombi, E.: A radio-isotopic dilution technique for functional characterisation of the associations between inorganic contaminants and water-dispersible naturally occurring soil colloids, Environ. Chem., 10, 341–348, https://doi.org/10.1071/EN13020, 2013.
Tiessen, H., Bettany, J. R., and Stewart, J. W. B.: An improved method for the determination of carbon in soils and soil extracts by dry combustion, Commun. Soil Sci. Plant Anal., 12, 211–218, https://doi.org/10.1080/00103628109367142, 1981.
Tipping, E.: WHAMC – A chemical equilibrium model and computer code for waters, sediments, and soils incorporating a discrete site/electrostatic model of ion-binding by humic substances, Comput. Geosci., 20, 973–1023, https://doi.org/10.1016/0098-3004(94)90038-8, 1994.
Toenniessen, G., Adesina, A., and DeVries, J.: Building an Alliance for a Green Revolution in Africa, Ann. NY Acad. Sci., 1136, 233–242, https://doi.org/10.1196/annals.1425.028, 2008.
Tye, A. M., Young, S. D., Crout, N. M. J., Zhang, H., Preston, S., Barbosa-Jefferson, V. L., Davison, W., McGrath, S. P., Paton, G. I., Kilham, K., and Resende, L.: Predicting the activity of Cd2+ and Zn2+ in soil pore water from the radio-labile metal fraction, Geochim. Cosmochim. Ac., 67, 375–385, https://doi.org/10.1016/S0016-7037(02)01138-9, 2003.
Van Eynde, E., Weng, L., and Comans, R. N. J.: Boron speciation and extractability in temperate and tropical soils: A multi-surface modeling approach, Appl. Geochem., 123, 104797, https://doi.org/10.1016/j.apgeochem.2020.104797, 2020.
Wessells, K. R. and Brown, K. H.: Estimating the Global Prevalence of Zinc Deficiency: Results Based on Zinc Availability in National Food Supplies and the Prevalence of Stunting, PLOS ONE, 7, e50568, https://doi.org/10.1371/journal.pone.0050568, 2012.
Wood, S. A., Tirfessa, D., and Baudron, F.: Soil organic matter underlies crop nutritional quality and productivity in smallholder agriculture, Agr. Ecosyst. Environ., 266, 100–108, https://doi.org/10.1016/j.agee.2018.07.025, 2018.
Young, S. D., Zhang, H., Tye, A. M., Maxted, A., Thums, C., and Thornton, I.: Characterizing the availability of metals in contaminated soils. I. The solid phase: sequential extraction and isotopic dilution, Soil Use Manage., 21, 450–458, https://doi.org/10.1079/SUM2005348, 2005.
Zia, M. H., Ahmed, I., Bailey, E. H., Lark, R. M., Young, S. D., Lowe, N. M., Joy, E. J. M., Wilson, L., Zaman, M., and Broadley, M.: Site-specific factors influence the field performance of a Zn-biofortified wheat variety, Front. Sustain. Food Syst., 4, 135, https://doi.org/10.3389/fsufs.2020.00135, 2020.